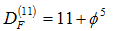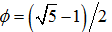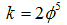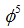Dark Energy Introduction

Thursday, December 26, 2013

Experimentally Based Theoretical Arguments that Unruh’s Temperature, Hawking’s Vacuum Fluctuation and Rindler’s Wedge Are Physically Real

Experimentally Based Theoretical Arguments that Unruh’s Temperature, Hawking’s Vacuum Fluctuation and Rindler’s Wedge Are Physically Real


Authors 
Mohamed S. El Naschie, Dept. of Physics, University of Alexandria, Egypt


To cite this article 
Mohamed S. El Naschie, Experimentally Based Theoretical Arguments that Unruh’s Temperature, Hawking’s Vacuum Fluctuation and Rindler’s Wedge Are Physically Real, American Journal of Modern Physics. Vol. 2, No. 6, 2013, pp. 357-361. doi: 10.11648/j.ajmp.20130206.23

Abstract 
The objective of the present paper is to argue that based on the reality of the observed increased rate of cosmic expansion, Unruh’s temperature, Hawking’s negative vacuum energy and Rindler’s wedge must also be a physical reality. We present first a brief derivation of the missing dark energy density of the universe which is in absolute agreement with the most recent accurate cosmological measurements and observations. The derivation is based upon a Rindler space setting, the associated wedge horizon and Unruh temperature. That way the topological ordinary energy is found to be half of the topological Unruh fluctuation mass m(O) = φ3 multiplied with the square of the topological speed of light c2 = φ2 where φ = 2 /(√5+ 1). This is exactly equal to the area of the spear-like hyperbolic triangular part of the Rindler wedge. The corresponding physical ordinary energy density is thus E(O) = (1/2)( φ3)( φ2) mc2 = (φ5/2)( mc2), where φ5 is Hardy’s probability of quantum entanglement. The topological dark energy density on the other hand is equal half of the topological Kaluza-Klein five dimensional mass m(D) = 5 multiplied with c2 = φ2. This in turn is exactly equal to the circular segment part of the wedge which together with the hyperbolic triangular entangled area forms the complete Lorentzian invariant triangular area of the wedge. Consequently the physical dark energy density which is uncorrelated, i.e. disentangled is given by E(D) = (1/2)(5)( φ2)( mc2) = (5 φ2 /2)( mc2) in full agreement with observation. Adding E(O) and E(D) one finds E(Einstein) = mc2 in full agreement with all our previous derivations. From the above we argue that since measurements, observations and theory have shown the increased expansion to be real and because the present derivation of the same results is based on Rindler’s space and Unruh’s temperature, it follows as a logical necessity that Unruh’s temperature, Hawking’s fluctuation and Rindler’s wedge are all physically real and can be measured, at least in principle.

Keywords 
Hawking Vacuum Fluctuation, Unruh Temperature, Rindler Wedge, Dark Energy, Quantum Gravity, Cantorian Spacetime, Hyperbolic Fractal Geometry

To continue reading the paper please downloads it from here

Reference 
[01]M.S. El Naschie: What is the missing dark energy in a nutshell and the Hawking-Hartle quantum wave collapse. Int. J. Astronomy & Astrophysics, Vol. 3, No. 3, 2013, pp. 205-211.
  
[02]L. Amendola and S. Tsujikawa: Dark Energy – Theory and Observations. Cambridge University Press, Cambridge (2010).
  
[03]S. Perlmutter et al: Supernova cosmology project collaboration. “Measurement of omega and lambda from 42 high-redshift supernova. The Astrophysical Journal, Vol. 517, No. 2, 1999, pp. 565-585.
  
[04]A.G. Riess et al: Observation evidence from supernova for an accelerating universe and cosmological constants. The Astronomical Journal. Vol. 116, P. 1009, 1998. Doi: 10.1086/300499.
  
[05]A.G. Riess et al: The farthest known supernova: Support for an accelerating universe and a glimpse of the epoch of deceleration. Astrophysical Journal, Vol. 560, 2001, pp. 49-71. Doi: 10.1086/322438.
  
[06]BP Schmidt et al: The high-Z supernova search: measuring cosmic deceleration and global curvature of the universe using type 1a supernovae. The Astrophysical Journal, Vol. 507, No. 1, 1998, pp. 46
  
[07]E.J. Copeland, M. Sami and S. Tsujikawa: Dynamics of dark energy, 2006. arXiv: hep-th/0603057V3.
  
[08]R. Panek: “Dark Energy”: The biggest mystery in the universe. 2010. http:/www.smithsonianmagazine.com/science.Nature/darkenergy.
  
[09]Planck-spacecraft. Wikipedia, 2012. http://en.wikipedia.org/wiki/Planck.
  
[10]M.S. El Naschie: A unified Newtonian-relativistic quantum resolution of the supposedly missing dark energy of the cosmos and the constancy of the speed of light. Int. J. Mod. Nonlinear Theory & Appli., Vol. 2, No. 1, 2013, pp. 55-59.
  
[11]L. Marek-Crnjac, M.S. El Naschie and Ji-Huan He: Chaotic fractals at the root of relativistic quantum physics and cosmology. Int. J. of Mod. Nonlinear Theory & Appli., Vol. 2, No. 1A, 2013, pp78-88.
  
[12]C. Toni: Dark matter, dark energy and the fate of Einstein’s theory of gravity. AMS Graduate Student Blog. Mathgrablog.williams,edu/dark-matter-darkenergy=fate-einstein-theory=gravity/
  
[13]M.S. El Naschie: Quantum entanglement: where dark energy and negative gravity plus accelerated expansion of the universe comes from. J. of Quant. Info. Sci., Vol. 3, 2013, pp. 57-77.
  
[14]WMAP Collaboration. E.Komatsu et al. “Seven Years Wilkinson Microwave Anisotropy probe (WMAP) Observations: Cosmological interpretation.” Astrophys. J. suppl 192 (2011) 18, arxiv: 1001.4538 [astro-ph.co].
  
[15]M.S. El Naschie: The quantum gravity Immirzi parameter – A general physical and topological interpretation. Gravitation and Cosmology, Vol. 19, No. 3, 2013, pp. 151-155.
  
[16]L. Susskind and J. Lindesay: Black holes, information and the string theory revolution. World Scientific, Singapore (2010).
  
[17]G. Ellis and R. Williams: Flat and Curved Space-Times. Oxford University Press, Oxford, 2000.
  
[18]W. Rindler: Relativity (Special, General and Cosmological). Oxford University press, Oxford. 2004.
  
[19]M.S. El Naschie: The hyperbolic extension of Sigalotti-Hendi-Sharif Zadeh’s golden triangle of special theory of relativity and the nature of dark energy. J. Mod. Phys., Vol. 4, No. 3, 2013, pp. 354-356.
  
[20]M.S. El Naschie and Atef Helal: Dark energy explained via the Hawking-Hartle quantum wave and the topology of cosmic crystallography. Int. J. Astron. & Astrophys, Vol. 3, No. 3, 2013, pp. 318-343.
  
[21]S. Hawking and G. Ellis: The Large Scale Structure of Spacetime. Cambridge University Press, Cambridge, 1973.
  
[22]S. Weinberg: Cosmology. Oxford University Press, Oxford (2008).
  
[23]Ta-Pei Cheng: Relativity, Gravitation and Cosmology. Oxford University Press, Oxford 2005.
  
[24]M.S. El Naschie: The missing dark energy of the cosmos from light cone topological velocity and scaling the Planck scale. Open J. of Microphysics, Vol. 3, No. 3, 2013, pp. 64-70.
  
[25]M.S. El Naschie: Topological-geometrical and physical interpretation of the dark energy of the cosmos as a “halo” energy of the Schrödinger quantum wave. J. Mod. Phys., Vol. 4, No. 5, 2013, pp. 591-596.
  
[26]G. Ford and R. O’Connell: Is there Unruh radiation? arXiv: quant-ph/0509151V 21 Septe. 2005.
  
[27]E. Akhmedov and D. Singleton: On the physical meaning of the Unruh effect. arXiv: 0705.2525V3[hep-th], 19 Oct. 2007.
  
[28]M.S. El Naschie and Ji-Huan He: The fractal geometry of quantum mechanics revealed. Fractal Spacetime and Noncommutative Geometry in High Energy Phys., Vol. 1, No. 1, 2011, pp. 3-9.
  
[29]A. Vilenkin: Effects of small-scale structure on the dynamics of cosmic strings. Phys. Rev. D, Vol. 41, No. 10, 1990, pp. 3038-3040.
  
[30]Yu N. Gnedin, A.A. Grib and V.M. Mostepanenko: Editors: Proc. of Third Alexander Friedmann Int. Seminar on Gravitation & Cosmology. Friedmann Lab. Publishers, St. Petersberg, 1995.
  
[31]A. Vilenkin and E. Shellard: Cosmic strings and other topological defects. Cambridge University Press, Cambridge, 2001.
  
[32]R. Penrose. The Road to Reality. Jonathan Cape. London (2004).
  
[33]J. Magueijo: Faster Than The Speed of Light. Arrow Books, The Random House, London (2003).
  
[34]V. Belinski, E. Verdaguer: Gravitational Solitons. Cambridge University Press, Cambridge, 2001.
  
[35]L.B. Okun: Energy and Mass in Relativity Theory. World Scientific, Singapore, 2009.
  
[36]M.S. El Naschie: Towards a general transfinite set theory for quantum mechanics. Fractal Spacetime and Noncommutative Geometry in High Energy Phys., Vol. 2, No. 2, 2012, pp. 135-142.
Posted by at No comments:

The Hydrogen Atom Fractal Spectra, the Missing Dark Energy of the Cosmos and Their Hardy Quantum Entanglement

The Hydrogen Atom Fractal Spectra, the Missing Dark Energy of the Cosmos and Their Hardy Quantum Entanglement

Author(s)
Mohamed S. El Naschie
In this letter, I outline the intimate connection between the fractal spectra of the exact solution of the hydrogen atom and the issue of the missing dark energy of the cosmos. A proposal for a dark energy reactor harnessing the dark energy of the Schrodinger wave via a quantum wave nondemolition measurement is also presented.
KEYWORDS
Fractal Spectra; Dark Energy; Golden Mean; KAM Theorem; Quantum Entanglement; Special Relativity


Cite this paper
M. Naschie, "The Hydrogen Atom Fractal Spectra, the Missing Dark Energy of the Cosmos and Their Hardy Quantum Entanglement," International Journal of Modern Nonlinear Theory and Application, Vol. 2 No. 3, 2013, pp. 167-169. doi: 10.4236/ijmnta.2013.23023.
[1]V. M. Petrusevski, “The H-Atom and the Golden Ratio: A Possible Link,” Journal of Chemical Education, Vol. 83, No. 1, 2006, p. 40.
[2]V. M. Petrusevski, “The First Excited State of the Hydrogen Atom and the Golden Ratio: A Link or a Mere Coincidence?” Bulletin of the Chemists and Technologists of Macedonia, Vol. 25, No. 1, 2006, pp. 61-63.
[3]C. L. Devito and W. A. Little, “Fractal Sets Associated with Function: The Spectral Lines of Hydrogen,” Physical Review A, Vol. 38, No. 12, 1988, pp. 6362-6364. doi:10.1103/PhysRevA.38.6362
[4]A. C. Phillips, “Introduction to Quantum Mechanics,” John Wiley & Sons Ltd., Chichester, 2003.
[5]M. S. El Naschie, “Quantum Entanglement as a Consequence of a Cantorian Micro Spacetime Geometry,” Journal of Quantum Information Science, Vol. 1, No. 2, 2011, pp. 50-53. doi:10.4236/jqis.2011.12007
[6]J.-H. He, et al., “Quantum Golden Mean Entanglement Test as the Signature of the Fractality of Micro Spacetime,” Nonlinear Science Letters B, Vol. 1, No. 2, 2011, pp. 45-50.
[7]L. Hardy, “Nonlocality of Two Particles without Inequalities for Almost All Entangled States,” Physical Review Letters, Vol. 71, No. 11, 1993, pp. 1665-1668. doi:10.1103/PhysRevLett.71.1665
[8]M. S. El Naschie, “A Review of E-Infinity Theory and the Mass Spectrum of High Energy Particle Physics,” Chaos, Solitons & Fractals, Vol. 19, No. 1, 2004, pp. 209-236. doi:10.1016/S0960-0779(03)00278-9
[9]M. S. El Naschie, “The Theory of Cantorian Spacetime and High Energy Particle Physics (An Informal Review),” Chaos, Solitons & Fractals, Vol. 41, No. 5, 2009, pp. 2635-2646. doi:10.1016/j.chaos.2008.09.059
[10]R. Penrose, “The Road to Reality,” Jonathan Cape, London, 2004.
[11]R. Mauldin and S. Williams, “Random Recursive Constructions: Asymptotic Geometries and Topological Properties,” Transactions of the American Mathematical Society, Vol. 295, No.1. 1986, pp. 325-346. doi:10.1090/S0002-9947-1986-0831202-5
[12]R. Mauldin, “On the Hausdorff Dimension of Graphs and Recursive Object,” In: G. Mayer-Kress, Ed., Dimension and Entropies in Chaotic Systems, Springer, Berlin, 1986, pp. 28-33.
[13]L. Marek-Crnjac, “The Hausdorff Dimension of the Penrose Universe,” Physics Research International, Vol. 2011, 2011, pp. 1-4.
[14]A. Connes, “Noncommutative Geometry,” Academic Press, San Diego, 1994.
[15]M. Gardener, “Penrose Tiles to Trapdoor Ciphers,” W.H. Freeman, New York, 1989.
[16]L. Amendola and S. Tsujikawa, “Dark Energy: Theory and Observations,” Cambridge University Press, Cambridge, 2010.
[17]L. Marek-Crnjac, et al., “Chaotic Fractals at the Root of Relativistic Quantum Physics and Cosmology,” International Journal of Modern Nonlinear Theory and Application, Vol. 2, No. 1A, 2013, pp. 78-88. doi:10.4236/ijmnta.2013.21A010
[18]M. S. El Naschie, “A Resolution of Cosmic Dark Energy via a Quantum Entanglement Relativity Theory,” Journal of Quantum Information Science, Vol. 3, No. 1, 2013, pp. 23-26. doi:10.4236/jqis.2013.31006
[19]M. S. El Naschie, “Topological-Geometrical and Physical Interpretation of the Dark Energy of the Cosmos as a ‘Halo’ Energy of the Schrodinger Quantum Wave,” Journal of Modern Physics, Vol. 4, No. 5, 2013, pp. 591-596. doi:10.4236/jmp.2013.45084
[20]S. Brandt and H. Dahmen, “The Picture Book of Quantum Mechanics,” Springer, New York, 1995, pp. 237-238.
Posted by at No comments:

Dark Energy Explained via the Hawking-Hartle Quantum Wave and the Topology of Cosmic Crystallography

Dark Energy Explained via the Hawking-Hartle Quantum Wave and the Topology of Cosmic Crystallography
Author(s)
Mohamed S. El Naschie, Atef Helal
The aim of the present paper is to explain and accurately calculate the missing dark energy density of the cosmos by scaling the Planck scale and using the methodology of the relatively novel discipline of cosmic crystallography and Hawking-Hartle quantum wave solution of Wheeler-DeWitt equation. Following this road we arrive at a modified version of Einstein’s energy mass relation E = mc2 which predicts a cosmological energy density in astonishing accord with the WMAP and supernova measurements and analysis. We develop non-constructively what may be termed super symmetric Penrose fractal tiling and find that the isomorphic length of this tiling is equal to the self affinity radius of a universe which resembles an 11 dimensional Hilbert cube or a fractal M-theory with a Hausdorff dimension where. It then turns out that the correct maximal quantum relativity energy-mass equation for intergalactic scales is a simple relativistic scaling, in the sense of Weyl-Nottale, of Einstein’s classical equation, namely EQR = (1/2)(1/) moc2 = 0.0450849 mc2 and that this energy is the ordinary measurable energy density of the quantum particle. This means that almost 95.5% of the energy of the cosmos is dark energy which by quantum particle-wave duality is the absolute value of the energy of the quantum wave and is proportional to the square of the curvature of the curled dimension of spacetime namely where and is Hardy’s probability of quantum entanglement. Because of the quantum wave collapse on measurement this energy cannot be measured using our current technologies. The same result is obtained by involving all the 17 Stein spaces corresponding to 17 types of the wallpaper groups as well as the 230-11=219 three dimensional crystallographic group which gives the number of the first level of massless particle-like states in Heterotic string theory. All these diverse subjects find here a unified view point leading to the same result regarding the missing dark energy of the universe, which turned out to by synonymous with the absolute value of the energy of the Hawking-Hartle quantum wave solution of Wheeler-DeWitt equation while ordinary energy is the energy of the quantum particle into which the Hawking-Hartle wave collapse at cosmic energy measurement. In other words it is in the very act of measurement which causes our inability to measure the “Dark energy of the quantum wave” in any direct way. The only hope if any to detect dark energy and utilize it in nuclear reactors is future development of sophisticated quantum wave non-demolition measurement instruments.
KEYWORDS
Doubly Special Relativity; Week’s Manifold; Experimental Test of Einstein’s Relativity; Witten’s M-Theory; Ordinary Energy of the Quantum Particle; Hawking-Hartle Wave of Cosmos; Crystallographic Symmetry Groups; Revising Special Relativity


Cite this paper
M. Naschie and A. Helal, "Dark Energy Explained via the Hawking-Hartle Quantum Wave and the Topology of Cosmic Crystallography," International Journal of Astronomy and Astrophysics, Vol. 3 No. 3, 2013, pp. 318-343. doi: 10.4236/ijaa.2013.33037.
[1]L. Amendola and S. Tsujikawa, “Dark Energy: Theory and Observations,” Cambridge University Press, Cambridge, 2010. doi:10.1017/CBO9780511750823
[2]Y. Baryshev and P. Teerikorpi, “Discovery of Cosmic Fractals,” World Scientific, Singapore, 2002.
[3]L. Nottale, “Scale Relativity,” Imperial College Press, London, 2011.
[4]R. Penrose, “The Road to Reality,” Jonathan Cape, London, 2004.
[5]J. Mageuijo and L. Smolin, “Lorentz Invariance with an Invariant Energy Scale,” Physical Review Letters, Vol. 88, No. 19, 2002, Article ID: 190403.
[6]J.-P. Luminet, “The Wraparound Universe,” A.K. Peters Ltd., Wellesley, 2008.
[7]M. S. El Naschie, “The Crystallographic Space Groups and Heterotic String Theory,” Chaos, Solitons & Fractals, Vol. 41, No. 5, 2009, pp. 2282-2284. doi:10.1016/j.chaos.2008.09.001
[8]J. R. Weeks, “The Shape of Space,” Marcel Dekker, New York, 2002.
[9]L. Marek-Crnjac, “The Hausdorff Dimension of the Penrose Universe,” Physics Research International, Vol. 2011, 2011, Article ID: 874302.
[10]I. Aitchison, “Super Symmetry in Particle Physics,” Cambridge University Press, Cambridge, 2007.
[11]A. Elokaby, “The Deep Connection between Instantons and String States Encoded in Klein’s Modular Space,” Chaos, Solitons & Fractals, Vol. 42, No. 1, 2009, pp. 303-305. doi:10.1016/ j.chaos.2008.12.001
[12]M. S. El Naschie, “Anomalies Free E-Infinity from von Neumann’s Continuous Geometry,” Chaos, Solitons & Fractals, Vol. 38, No. 5, 2008, pp. 1318-1322. doi:10.1016/j.chaos.2008.06.025
[13]M. S. El Naschie, “Quasi Exceptional E12 Lie Symmetry Group with 685 Dimensions, KAC-Moody Algebra and E-Infinity Cantorian Spacetime,” Chaos, Solitons & Fractals, Vol. 38, No. 4, 2008, pp. 990-992. doi:10.1016/j.chaos.2008.06.015
[14]M. S. El Naschie, “Average Exceptional Lie and Coxeter Group Hierarchies with Special Reference to the Standard Model of High Energy Particle Physics,” Chaos, Solitons & Fractals, Vol. 37, No. 3, 2008, pp. 662-668. doi:10.1016/j.chaos.2008.01.018
[15]M. S. El Naschie, “KAC-Moody Exceptional E12 from Simplictic Tiling,” Chaos, Solitons & Fractals, Vol. 41, No. 4, 2009, pp. 1569-1571. doi:10.1016/j.chaos.2008.06.020
[16]M. S. El Naschie, “The Internal Dynamics of the Exceptional Lie Symmetry Groups Hierarchy and the Coupling Content of Unification,” Chaos, Solitons & Fractals, Vol. 38, No. 4, 2008, pp. 1031-1038.
[17]M. S. El Naschie, “High Energy Physics and the Standard Model from the Exceptional Lie Groups,” Chaos, Solitons & Fractals, Vol. 36, No. 1, 2008, pp. 1-17. doi:10.1016/j.chaos.2007.08.058
[18]M. S. El Naschie, “Symmetry Group Prerequisites for E-Infinity in High Energy Physics,” Chaos, Solitons & Fractals, Vol. 35, No. 1, 2008, pp. 202-211. doi:10.1016/j.chaos.2007.05.006
[19]M. S. El Naschie, “Fuzzy Knot Theory Interpretation of Yang-Mills Instantons and Witten’s 5-Brane Model,” Chaos, Solitons & Fractals, Vol. 38, No. 5, 2008, pp. 1349-1354. doi:10.1016/ j.chaos.2008.07.002
[20]J.-H. He, “Hilbert Cube Model for Fractal Spacetime,” Chaos, Solitons & Fractals, Vol. 42, No. 5, 2009, pp. 2754-2759. doi:10.1016/j.chaos.2009.03.182
[21]M. S. El Naschie, “An Irreducibly Simple Derivation of the Hausdorff Dimension of Spacetime,” Chaos, Solitons & Fractals, Vol. 41, No. 5, 2009, pp. 1902-1904. doi:10.1016/j.chaos.2008.07.043
[22]M. S. El Naschie, “A Review of E-Infinity and the Mass Spectrum of High Energy Particle Physics,” Chaos, Solitons & Fractals, Vol. 19, No. 1, 2004, pp. 209-236. doi:10.1016/S0960-0779(03)00278-9
[23]M. S. El Naschie, “On an Eleven Dimensional E-Infinity Fractal Spacetime Theory,” International Journal of Nonlinear Sciences and Numerical Simulation, Vol. 7, No. 4, 2006, pp. 407-409.
[24]M. S. El Naschie, “The Discrete Charm of Certain Eleven Dimensional Spacetime Theory,” International Journal of Nonlinear Sciences and Numerical Simulation, Vol. 7, No. 4, 2006, pp. 477-481.
[25]M. S. El Naschie, “The Theory of Cantorian Spacetime and High Energy Particle Physics (An Informal Review),” Chaos, Solitons & Fractals, Vol. 41, No. 5, 2009, pp. 2635-2646. doi:10.1016/ j.chaos.2008.09.059
[26]J.-H. He, et al., “Quantum Golden Mean Entanglement Test as the Signature of the Fractality of Micro Spacetime,” Nonlinear Science Letters B, Vol. 1, No. 2, 2011, pp. 45-50.
[27]M. S. El Naschie, “Quantum Entanglement as a Consequence of a Cantorian Micro Spacetime Geometry,” Journal of Quantum Information Science, Vol. 1, No. 2, 2011, pp. 50-53. doi:10.4236/ jqis.2011.12007
[28]M. S. El Naschie, “Derivation of the Euler Characteristic and Curvature of Cantorian Fractal Spacetime Using Nash Euclidean Embedding and the Universal Menger Sponge,” Chaos, Solitons & Fractals, Vol. 41, No. 5, 2009, pp. 2394-2398. doi:10.1016/j.chaos.2008.09.021
[29]M. S. El Naschie, “Arguments for Compactness and Multiple Connectivity of Our Cosmic Spacetime,” Chaos, Solitons & Fractals, Vol. 41, No. 5, 2009, pp. 2787-2789. doi:10.1016/j.chaos.2008.10.011
[30]M. S. El Naschie, “Light Cone Quantization, Heterotic Strings and E-Infinity Derivation of the Number of Higgs Bosons,” Chaos, Solitons & Fractals, Vol. 23, No. 5, 2005, pp. 1931-1933. doi:10.1016/ j.chaos.2004.08.004
[31]M. S. El Naschie, “Fuzzy Platonic Spaces as a Model for Quantum Physics,” Mathematical Models, Physical Methods and Simulation in Science & Technology, Vol. 1, No. 1, 2008, pp. 69-90.
[32]M. S. El Naschie, “Hilbert Space, Poincaré Dodecahedron and Golden Mean Transfiniteness,” Chaos, Solitons & Fractals, Vol. 31, No. 4, 2007, pp. 787-793. doi:10.1016/j.chaos.2006.06.003
[33]M. S. El Naschie, “Transfinite Harmonization by Taking the Dissonance Out of the Quantum Field Symphony,” Chaos, Solitons & Fractals, Vol. 36, No. 4, 2008, pp. 781-786. doi:10.1016/ j.chaos.2007.09.018
[34]S. Nakajima, et al., “Foundations of Quantum Mechanics in the Light of New Technologies,” World Scientific, Singapore, 1996.
[35]W. Rindler, “Relativity (Special, General and Cosmological),” Oxford University Press, Oxford, 2004.
[36]G. W. Gibbons, et al., “The Future of Theoretical Physics and Cosmology,” Cambridge University Press, Cambridge, 2003.
[37]S. Hawking, et al., “Brane New World,” Physical Review D, Vol. 62, No. 4, 2000, Article ID: 043501. doi:10.1103/PhysRevD.62.043501
[38]M. S. El Naschie, “A Unified Newtonian-Relativistic Quantum Resolution of the Supposedly Missing Dark Energy of the Cosmos and the Constancy of the Speed of Light,” International Journal of Modern Nonlinear Theory and Application, Vol. 2, No. 1, 2013, pp. 43-54. doi:10.4236/ ijmnta.2013.21005
[39]L. Marek-Crnjac, et al., “Chaotic Fractals at the Root of Relativistic Quantum Physics and Cosmology,” International Journal of Modern Nonlinear Theory and Application, Vol. 2, No. 1A, 2013, pp. 78-88.
[40]M. S. El Naschie, “The Hyperbolic Extension of Sigalotti-Hendi-Sharifzadeh’s Golden Triangle of Special Theory of Relativity and the Nature of Dark Energy,” Journal of Modern Physics, Vol. 4, No. 3, 2013, pp. 354-356. doi:10.4236/jmp.2013.43049
[41]M. S. El Naschie, S. Olsen, J. H. He, S. Nada, L. Marek-Crnjac, A. Helal, et al., “On the Need for Fractal Logic in High Energy Quantum Physics,” International Journal of Modern Nonlinear Theory and Application, Vol. 1, No. 3, 2012, pp. 84-92. doi:10.4236/ijmnta.2012.13012
[42]J. Guckenheimer and P. Holmes, “Nonlinear Dynamical Systems and Bifurcation of Vector Fields,” Springer Verlag, New York, 1994.
[43]P. S. Wesson, “Five-Dimensional Physics,” World Scientific, Singapore, 2006.
[44]F. Morgan, “Geometric Measure Theory,” Academic Press, Amsterdam, 2009.
[45]M. S. El Nasche, “A Fractal Menger Sponge Spacetime Proposal to Reconcile Measurements and Theoretical Predictions of Cosmic Dark Energy,” International Journal of Modern Nonlinear Theory and Application, Vol. 2, No. 2, 2013, pp. 107-121.
[46]M. S. El Naschie, “Quantum Entanglement: Where Dark Energy and Negative Gravity plus Accelerated Expansion of the Universe Comes from,” Journal of Quantum Information Science, Vol. 3, No. 2, 2013, pp. 57-77. doi:10.4236/jqis.2013.32011
[47]M. S. El Naschie, “Dark Energy from Kaluza-Klein Spacetime and Noether’s Theorem via Lagrangian Multiplier Method,” Journal of Modern Physics, Vol. 4, No. 6, 2013, pp. 757-760. doi:10.4236/ jmp.2013.46103
[48]M. S. El Naschie, “Elementary Prerequisites for E-Infinity,” Chaos, Solitons & Fractals, Vol. 30, No. 3, 2006, pp. 579-605. doi:10.1016/j.chaos.2006.03.030
[49]B. Kosko, “Fuzzy Thinking,” Flamingo-Harper Collins Publishers, London, 1993.
[50]M. S. El Naschie, “Is Einstein’s General Field Equation More Fundamental than Quantum Mechanics and Particle Physics,” Chaos, Solitons & Fractals, Vol. 30, No. 3, 2006, pp. 525-531. doi:10.1016/ j.chaos.2005.04.123
[51]P. Halpern, “The Great Beyond,” John Wiley & Sons, New York, 2004.
[52]E. J. Copeland, M. Sami and S. Tsujikawa, “Dynamics of Dark Energy,” International Journal of Modern Physics D, Vol. 15, No. 11, 2006, pp. 1753-1936.
[53]J. Magueijo and J. W. Moffat, “Comments on ‘Note on Varying Speed of Light Theories’,” General Relativity and Gravitation, Vol. 40, 2007, pp. 1797-1803.
[54]J. Magueijo and L. Smolin, “Gravity’s Rainbow,” Classical and Quantum Gravity, Vol. 21, No. 7, 2004, p. 1725.
[55]R. Calella, A. Woverhauser and S. A. Werner, “Observation of Gravitationally Induced Quantum Interference,” Physical Review Letters, Vol. 34, No. 23, 1975, pp. 1472-1474. doi:10.1103/ PhysRevLett.34.1472
[56]M. S. El Naschie, “A Resolution of the Cosmic Dark Energy via Quantum Entanglement Relativity Theory,” Journal of Quantum Information Science, Vol. 3, No. 1, 2013, pp. 23-26. doi:10.4236/ jqis.2013.31006
[57]V. V. Nesvizhevky and A. K. Petukhov, “Study of Neutron Quantum States in the Gravity Field,” European Physical Journal C, Vol. 40, No. 4, 2005, p. 479. doi:10.1140/epjc/s2005-02135-y
[58]D. Gross, “Can We Scale the Planck Scale?” Physics Today, Vol. 42, No. 6, 1989, p. 9.
Posted by at No comments:

Chaotic Fractal Tiling for the Missing Dark Energy and Veneziano Model

Chaotic Fractal Tiling for the Missing Dark Energy and Veneziano Model
Author(s)
L. Marek-Crnjac, M. S. El Naschie
The formula for the quantum amplitude of the Veneziano dual resonance model is shown to be formally analogous to the dimensionality of a K-theoretical fractal quotient manifold of the non-commutative geometrical type. Subsequently this analogy is used to deduce the ordinary energy of the quantum particle and the dark energy of the quantum wave. The results agree completely with cosmological measurements. Even more surprisingly the sum of both energy expressions turned out to be exactly equal to Einstein’s iconic formula E = mc2. Consequently Einstein’s formula makes no distinction between ordinary and dark energy.
KEYWORDS
Hausdorff Dimension; Cantor Set; Dark Energy; Kähler Manifold; Quantum Entanglement; Veneziano Model


Cite this paper
L. Marek-Crnjac and M. Naschie, "Chaotic Fractal Tiling for the Missing Dark Energy and Veneziano Model,"Applied Mathematics, Vol. 4 No. 11B, 2013, pp. 22-29. doi: 10.4236/am.2013.411A2005.
[1]L. Amendola and S. Tsujikawa, “Dark Energy, Theory and Observations,” Cambridge University Press, Cambridge, 2010.
[2]B. Carr, “Universe or Multiverse?” Cambridge University Press, Cambridge, 2010.
[3]Y. Baryshev and P. Teerikorpi, “Discovery of Cosmic Fractals,” World Scientific, Singapore, 2011.
[4]L. Nottale, “Scale Relativity,” Imperial College Press, London, 2011.
[5]G. Barenblatt, “Scaling,” Cambridge University Press, Cambridge, 2003.
http://dx.doi.org/10.1017/CBO9780511814921
[6]J. Mageuijo and L. Smolin, “Lorentz Invariance with an Invariant Energy Scale,” arXiv: hep-th/0112090V2, 18 December 2001.
[7]G. Veneziano, “Ward Identities in Dual String Theories,” Physics Letters B, Vol. 167, No. 4, 1986, pp. 388-392.
[8]Y. Nambu, “Quark Model and the Factorization of Veneziano Amplitude, In: R. Choud, Ed., Symmetries and Quark Models, Gordon and Breach, New York, 1970, pp. 269-278.
[9]V. Vladimirov. I. Valovich and E. Zelenov, “P-Adic Analysis and Mathematical Physics,” World Scientific, Singapore, 1994. http://dx.doi.org/10.1142/1581
[10]A. Connes, “Non-Commutative Geometry,” Academic Press, San Diego, 1994.
[11]R. Penrose, “The Road to Reality,” Jonathan Cape, London, 2004.
[12]L. Hardy, “Non-Locality of Two Particles without Inequalities for Almost All Entangled States,” Physical Review Letters, Vol. 71, No. 11, 1993, pp. 1665-1668.
http://dx.doi.org/10.1103/PhysRevLett.71.1665
[13]C. Nash and S. Sen, “Topology and Geometry for Physicists,” Academic Press, San Diego, 1983.
[14]I. Buchbinder, S. Odintsov and I. Shapiro, “Effective Action in Quantum Gravity,” Institute of Physics Publishing, Bristol, 1992.
[15]M. Green, J. Schwarz and E. Witten, “Superstring Theory,” Cambridge University Press, Cambridge, 1987.
[16]M. Kaku, “Introduction to Superstrings and M-Theory,” Springer, New York, 1999.
http://dx.doi.org/10.1007/978-1-4612-0543-2
[17]J.-H. He, “Hilbert Cube Model for Fractal Space-Time,” Chaos, Solitons & Fractals, Vol. 42, No. 5, 2009, pp. 2754-2759. http://dx.doi.org/10.1016/j.chaos.2009.03.182
[18]J.-H. He, “Twenty Six Dimensional Polytope and High Energy Spacetime Physics,” Chaos, Solitons & Fractals, Vol. 33, No. 1, 2007, pp. 5-13.
http://dx.doi.org/10.1016/j.chaos.2006.10.048
[19]D. Joyce, “Compact Manifold with Special Holonom,” Oxford Press, Oxford, 2003.
[20]T. Hübsch, “Calabi-Yau Manifolds,” World Scientific, Singapore, 1994.
[21]E. Charpentier, A. Lesne and N. Nikolski, “Kolmogorov’s Heritage in Mathematics,” Springer, Berlin, 2007.
[22]M. S. El Naschie, “Quantum Entanglement: Where Dark Energy and Negative Gravity plus Accelerated Expansion of the Universe Comes From,” Journal of Quantum Information Science, Vol. 3, No. 2, 2013, pp. 57-77.
http://dx.doi.org/10.4236/jqis.2013.32011
[23]M. S. El Naschie, “The Missing Dark Energy of the Cosmos From Light Cone Topological Velocity and Scaling the Planck Scale,” Open Journal of Microphysics, Vol. 3, No. 3, 2013, pp. 64-70.
http://dx.doi.org/10.4236/ojm.2013.33012
[24]M. S. El Naschie and A. Helal, “Dark Energy Explained via the Hawking-Hartle Quantum Wave and the Topology of Cosmic Crystallography,” International Journal of Astronomy and Astrophysics, Vol. 3, No. 3, 2013, pp. 318343.
[25]M. S. El Naschie, “The Quantum Gravity Immirzi Parameter—A General Physical and Topological Interpretation,” Gravitation and Cosmology, Vol. 19, No. 3, 2013, pp. 151-155.
http://dx.doi.org/10.1134/S0202289313030031
[26]M. S. El Naschie, “What Is the Missing Dark Energy in a Nutshell and the Hawking-Hartle Quantum Wave Collapse,” International Journal of Astronomy and Astrophysics, Vol. 3, No. 3, 2013, pp. 205-211.
http://dx.doi.org/10.4236/ijaa.2013.33024
[27]L. Marek-Crnjac, “Modification of Einstein’s E = mc2 to E = mc2/22,” American Journal of Modern Physics, Vol. 2, No. 5, 2013, pp. 255-263.
[28]M. S. El Naschie, “A Resolution of the Cosmic Dark Energy via a Quantum Entanglement Relativity Theory,” Journal of Quantum Information Science, Vol. 3, No. 1, 2013, pp. 23-26.
http://dx.doi.org/10.4236/jqis.2013.31006
[29]M. S. El Naschie, “Dark Energy from Kaluza-Klein Spacetime and Noether’s Theorem via Lagrangian Multiplier Method,” Journal of Modern Physics, Vol. 4, No. 6, 2013, pp. 757-760.
http://dx.doi.org/10.4236/jmp.2013.46103
[30]M. S. El Naschie, “Determining the Missing Dark Energy of the Cosmos from a Light Cone Exact Relativistic Analysis,” Journal of Modern Physics, Vol. 2, No. 2, 2013, pp. 18-23.
[31]M. S. El Naschie, “Towards a General Transfinite Set Theory for Quantum Mechanics,” Fractal Space-Time and Non-Commutative Geometry in High Energy Physics, Vol. 2, No. 2, 2012, pp. 135-142.
[32]R. Elwes, “Ultimate Logic,” New Scientist, Vol. 211, No. 2823, 2011, pp. 30-33.
http://dx.doi.org/10.1016/S0262-4079(11)61838-1
[33]V. Jacques, et al., “Delayed-Choice Test of Quantum Complementarity with Interfering Single Photons,” Physical Review Letters, Vol. 100, No. 22, 2008, Article ID: 220402.
http://dx.doi.org/10.1103/PhysRevLett.100.220402
[34]L. Li, N. L. Liu and Z. X. Yu, “Duality Relations in a Two Path Interferometer with an Asymmetric Beam Splitter,” Physical Review A, Vol. 85, No. 5, 2012, Article ID: 054101.
http://dx.doi.org/10.1103/PhysRevA.85.054101
[35]M. F. Schriber, “Another Step Back for Wave-Particle Duality,” Physics, Vol. 4, No. 102, 2011, Article ID: 230406.
[36]J.-S. Tang et al., “Revisiting Bohr’s Principle of Complementarity Using a Quantum Device,” arXiv: 1204.5304V1[quant-ph], 24 April 2012.
[37]T. Jacobson, et al., “Increase of Black Hole Entropy in Higher Curvature Gravity,” arXiv: gr-qc/9503020V1, 11 March 1995.
[38]V. Vedral, “In from the Cold,” New Scientist, Vol. 216, No. 2886, 2012, pp. 33-37.
[39]L. M. Krauss, “A Higgs-Saw Mechanism as a Source of Dark Energy,” arXiv:1306.3239V1[hep-ph], 13 June 2013.
[40]L. Grossman, “Dark Energy May Spring from the Higgs Boson,” New Scientist, Vol. 219, No. 2931, 2013, p. 11.
http://dx.doi.org/10.1016/S0262-4079(13)62067-9
[41]D. Mermin, “Quantum Mechanics: Fixing the Shifty Split,” Physics Today, Vol. 65, No. 7, 2012, pp. 8-10.
http://dx.doi.org/10.1063/PT.3.1618
[42]M. S. El Naschie, “A Note on Quantum Gravity and Cantorian Spacetime,” Chaos, Solitons & Fractal, Vol. 8, No. 1, 1997, pp. 131-133.
http://dx.doi.org/10.1016/S0960-0779(96)00128-2
[43]M. S. El Naschie, “Complex Vacuum Fluctuation as a Chaotic ‘Limit’ Set of Any Kleinian Group Transformation and the Mass Spectrum of High Energy Particle Physics via Spontaneous Self-Organization,” Chaos, Solitons & Fractals, Vol. 17, No. 4, 2003, pp. 631-638.
http://dx.doi.org/10.1016/S0960-0779(02)00630-6
[44]M. S. El Naschie, “VAK, Vacuum Fluctuation and the Mass Spectrum of High Energy Particle Physics,” Chaos, Solitons & Fractals, Vol. 17, No. 4, 2003, pp. 797-807.
http://dx.doi.org/10.1016/S0960-0779(02)00684-7
[45]M. S. El Naschie, “The VAK of Vacuum Fluctuation, Spontaneous Self-Organization and Complexity Theory Interpretation of High Energy Particle Physics and the Mass Spectrum,” Chaos, Solitons & Fractals, Vol. 18, No. 2, 2003, pp. 401-420.
http://dx.doi.org/10.1016/S0960-0779(03)00098-5
[46]J.-H. He, “A Note on Elementary Cobordism and Negative Space,” International Journal of Nonlinear Sciences and Numerical Simulation, Vol. 11, No. 12, 2010, pp. 1093-1095.
[47]M. S. El Naschie, “Average Symmetry, Stability and Ergodicity of Multidimensional Cantor Sets,” Il Nuovo Cimento, Vol. 109, No. 2, 1994, pp. 149-157.
http://dx.doi.org/10.1007/BF02727425
[48]M. S. El Naschie, “Mathematical Foundations of E-Infinity via Coxeter and Reflection Groups,” Chaos, Solitons & Fractals, Vol. 37, No. 5, 2008, pp. 1267-1268.
http://dx.doi.org/10.1016/j.chaos.2008.02.001
[49]M. S. El Naschie, “Removing Spurious Non-Linearity in the Structure of Micro-Space-Time and Quantum Field Renormalization,” Chaos, Solitons & Fractals, Vol. 37, No. 1, 2008, pp. 60-64.
http://dx.doi.org/10.1016/j.chaos.2007.10.005
[50]M. S. El Naschie, “On ’t Hooft Dimensional Regularization in E-Infinity Space,” Chaos, Solitons & Fractals, Vol. 12, No. 5, 2001, pp. 851-858.
http://dx.doi.org/10.1016/S0960-0779(00)00138-7
[51]O. E. Rossler, et al., “Hubble Expansion in Static SpaceTime,” Chaos, Solitons & Fractals, Vol. 33, No. 3, 2007, pp. 770-775.
http://dx.doi.org/10.1016/j.chaos.2006.06.046
[52]M. Pusey, J. Barrett and T. Randolph, “On the Reality of Quantum State,” Nature Physics, Vol. 8, June 2012, pp. 475-478.
[53]M. S. El Naschie, “Mohamed El Naschie Answers a Few Questions about This Month’s Emerging Research Front in the Field of Physics,” 2004.
http://esi-topics.com/erf/2004/october04-MohamedElNaschie.html
[54]M. S. El Naschie, “This Month’s New Hot Paper in the Field of Engineering: On a Fuzzy Kahler-Like Manifold Which Is Consistent with the Two Slit Experiment,” International Journal of Nonlinear Sciences and Numerical Simulation, Vol. 6, No. 2, 2005, pp. 95-98.
http://esi-topics.com/nhp/2006/september-06-MohamedElNaschie.html
[55]M. Persinger and C. Lavellee, “Theoretical and Experimental Evidence of Macroscopic Entanglement between Human Brain Activity and Photon Emission,” Journal of Consciousness Exploration & Research, Vol. 1, No. 7, 2010, pp. 785-807.
[56]M. S. El Naschie, “COBE Satellite Measurement, Cantorian Space and Cosmic Strings,” Chaos, Solitons & Fractals, Vol. 8, No. 5, 1977, pp. 847-850.
http://dx.doi.org/10.1016/S0960-0779(97)00084-2
[57]L. Marek-Crnjac, “The Physics of Empty Sets and the Quantum,” Nonlinear Science Letters B, Vol. 1, No. 1, 2011, pp. 13-14.
[58]J.-H. He, “The Importance of the Empty Set Underpinning the Foundation of Quantum Physics,” Nonlinear Science Letters B, Vol. 1, No. 1, 2011, pp. 6-7.
[59]M. S. El Naschie, “Penrose Universe and Cantorian Spacetime as a Model for Noncommutative Quantum Geometry,” Chaos, Solitons & Fractals, Vol. 9, No. 6, 1998, pp. 931-933.
http://dx.doi.org/10.1016/S0960-0779(98)00077-0
[60]M. S. El Naschie, “Stress, Stability and Chaos in Structural Engineering,” McGraw Hill, London, 1990.
[61]D. Horrockos and W. Johnson, “On Anticlastic Curvature with Special Reference to Plastic Bending,” The International Journal of Mechanical Sciences, Vol. 9, No. 12, 1967, pp. 835-861.
http://dx.doi.org/10.1016/0020-7403(67)90011-2
[62]E. Cosserat and F. Cosserat, “Theorie des Corps Deformables,” Lavoisier S.A.S., Paris, 1909.
[63]M. S. El Naschie, “A Fractal Menger Sponge Spacetime Proposal to Reconcile Measurements and Theoretical Predictions of Cosmic Dark Energy,” International Journal of Modern Nonlinear Theory and Application, Vol. 2, No. 2, 2013, pp. 107-121.
http://dx.doi.org/10.4236/ijmnta.2013.22014
[64]M. S. El Naschie, “On the Philosophy of Being and Nothingness in Fundamental Physics,” Nonlinear Science Letters A, Vol. 2, No. 1, 2011, pp. 5-6.
[65]M. S. El Naschie, “On the Mathematical Philosophy of Being and Nothingness in Quantum Physics,” Fractal Space-Time & Non-Commutative Geometry in Quantum and High Energy Physics, Vol. 2, No. 2, 2012, pp. 103106.
Posted by at No comments:

Nash Embedding of Witten’s M-Theory and the Hawking-Hartle Quantum Wave of Dark Energy

Nash Embedding of Witten’s M-Theory and the Hawking-Hartle Quantum Wave of Dark Energy
Author(s)
Mohamed S. El Naschie
Euclidean embedding of the 11-dimensional M-theory turned out to require a very large space leaving lavish amounts of 242 dimensional pseudo truly empty “regions” devoid of space and time and consequently of anything resembling ordinary physical energy density. It is shown here using Nash embedding that the ratio of “solid” M-theory spacetime to its required embedding “non-spacetime” is 1/22 for a classical theory and1/22.18033989 for an analogous fractal theory. This then leads to a maximal ordinary energy density equation equal to that of Einstein’s famous formula E=mc2 but multiplied with  in full agreement with previous results obtained using relatively more conventional methods including running the electromagnetic fine structure constant in the exact solution of the hydrogen atom. Consequently, the new equation corresponds to a quantum relativity theory which unlike Einstein’s original equation gives quantitative predictions which agree perfectly with the cosmological measurements of WMAP and the analysis of certain supernova events. Never the less in our view dark energy also exists being the energy of the quantum wave amounting to 95.5 present of the total Einstein theoretical energy which is blind to any distinction between ordinary energy of the quantum particle and the dark energy of the quantum wave. However, since measurement leads to the collapse of the Hawking-Hartle quantum wave, dark energy being a quantum wave non-ordinary energy could not possibly be measured in the usual way unless highly refined quantum wave non-demolition technology is developed if possible. It is a further reason that dark energy having a different sign to ordinary energy is the cause behind the anti gravity force which is pushing the universe apart and accelerating cosmic expansion. Consequently it can be seen as the result of anticlastic Cartan-like curvature caused by extra compactified dimensions of spacetime. A simple toy model demonstration of the effect of curvature in a “material” space is briefly discussed.
KEYWORDS
Nash Euclidean Embedding; Quantum Entanglement; Dark Energy of the Quantum Wave; Quantum Gravity; Ordinary Energy of the Quantum Particle; Hawking-Hartle Wave of the Cosmos; Quantum Wave Non-Demolition; Witten’s M-Theory



Cite this paper
M. Naschie, "Nash Embedding of Witten’s M-Theory and the Hawking-Hartle Quantum Wave of Dark Energy," Journal of Modern Physics, Vol. 4 No. 10, 2013, pp. 1417-1428. doi: 10.4236/jmp.2013.410170.
[1]J. Nash, Annals of Mathematics, Vol. 63, 1956, p. 20.
http://dx.doi.org/10.2307/1969989
[2]J. Nash, Koninklijke Nederlandse Akademie van Wetenschappen, Vol. 58, 1955, p. 545.
[3]M. S. El Naschie, Chaos, Solitons & Fractals, Vol. 18, 2003, pp. 635-641.
http://dx.doi.org/10.1016/S0960-0779(03)00007-9
[4]R. Penrose, “The Road to Reality. A Complete Guide to the Laws of the Universe,” Jonathan Cape, London, 2004.
[5]W. Rindler, “Relativity,” Oxford Science Publications, Oxford, 2004.
[6]M. S. El Naschie and L. Marek-Crnjac, International Journal of Modern Nonlinear Theory and Application, Vol. 1, 2012, pp. 118-124.
http://dx.doi.org/10.4236/ijmnta.2012.14018
[7]E. J. Copeland, M. Sami and S. Tsujikawa, “Dynamics of Dark Energy,” 2006. arXiv: hep-th/0603057
[8]L. Amendola and S. Tsujikawa, “Dark Energy: Theory and Observations,” Cambridge University Press, Cambridge, 2010.
http://dx.doi.org/10.1017/CBO9780511750823
[9]M. S. El Naschie, Chaos, Solitons & Fractals, Vol. 41, 2009, p. 2635.
http://dx.doi.org/10.1016/j.chaos.2008.09.059
[10]M. S. El Naschie, M. S. El Naschie, Chaos, Solitons & Fractals, Vol. 19, 2004, p. 209.
http://dx.doi.org/10.1016/S0960-0779(03)00278-9
[11]M. S. El Naschie, Chaos, Solitons & Fractals, Vol. 30, 2006, p. 579.
http://dx.doi.org/10.1016/j.chaos.2006.03.030
[12]J. Polchinski, “String Theory,” Cambridge University Press, Cambridge, 1998.
[13]M. S. El Naschie, International Journal of Nonlinear Sciences and Numerical Simulation, Vol. 7, 2006, p. 477.
[14]M. S. El Naschie, International Journal of Nonlinear Sciences and Numerical Simulation, Vol. 7, 2006, p. 407.
[15]M. S. El Naschie, L. Marek-Crnjac and J.-H. He, Fractal Spacetime & Noncommutative Geometry in Quantum & High Energy Physics, Vol. 2, 2012, p. 107.
[16]M. Duff, International Journal of Modern Physics A, Vol. 11, 1996, p. 5623.
http://dx.doi.org/10.1142/S0217751X96002583
[17]L. Marek-Crnjac, J.-H. He and M. S. El Naschie, Fractal Spacetime & Noncommutative Geometry in Quantum & High Energy Physics, Vol. 2, 2012, p. 118.
[18]S. Nakajima, et al., “Foundations of Quantum Mechanics in The Light of New Technologies,” World Scientific, Singapore City, 1996.
[19]L. Marek-Crnjac, et al., International Journal of Modern Nonlinear Theory and Application, Vol. 2, 2013, p. 78.
[20]M.S. El Naschie, Journal of Quantum Information Science, Vol. 3, 2013, p. 23.
http://dx.doi.org/10.4236/jqis.2013.31006
[21]L. Marek-Crnjac and J.-H. He, Fractal Spacetime and Noncommutative Geometry in Quantum and High Energy Physics, Vol. 2, 2012, p. 66.
[22]V Braginsky and S. P. Vyatchanin, Doklady Akademii Nauk SSSR, Vol. 259, 1981, p. 570. [Soviet Physics— Doklady, Vol. 27, 1982, p. 478].
[23]M. S. El Naschie, Journal of Modern Physics, Vol. 4, 2013, p. 591. http://dx.doi.org/10.4236/jmp.2013.45084
[24]M. S. El Naschie, International Journal of Modern Nonlinear Theory and Application, Vol. 2, 2013, p. 43.
http://dx.doi.org/10.4236/ijmnta.2013.21005
[25]M.S. El Naschie, Journal of Quantum Information Science, Vol. 3, 2013, p. 57.
http://dx.doi.org/10.4236/jqis.2013.32011
[26]P. S. Wesson, “Five Dimensional Physics,” World Scientific, Singapore City, 2006.
[27]M. S. El Naschie, “Stress, Stability and Chaos in Structural Engineering,” McGraw Hill, London, 1990.
[28]M. S. El Naschie, International Journal of Modern Nonlinear Theory and Application, Vol. 2, 2013, pp. 107-121.
http://dx.doi.org/10.4236/ijmnta.2013.22014
[29]M. S. El Naschie, Chaos, Solitons & Fractals, Vol. 23, 2005, pp. 1511-1514.
http://dx.doi.org/10.1016/j.chaos.2004.08.008
[30]M. S. El Naschie, Chaos, Solitons & Fractals, Vol. 23, 2005, pp. 1931-1933.
http://dx.doi.org/10.1016/j.chaos.2004.08.004
[31]R. Calella, A. W. Overhauser and S. A. Werner, Physical Review Letters, Vol. 34, 1975, pp. 1472-1474.
http://dx.doi.org/10.1103/PhysRevLett.34.1472
[32]V. V. Nesvizhevky and A. K. Petukhov, The European Physical Journal C, Vol. 40, 2005, pp. 479-491.
http://dx.doi.org/10.1140/epjc/s2005-02135-y
[33]M. Reza Pahlavani, H. Rahbar and M. Ghezelbash, Open Journal of Microphysics, Vol. 3, 2013, pp. 1-7.
http://dx.doi.org/10.4236/ojm.2013.31001
[34]J. Magueijo and J. W. Moffat, “Comments on ‘Note on Varying Speed of Light Theories’,” 2007.
arXiv: 0705.4507
[35]J. Magueijo, “Faster Than the Speed of Light,” Arrow Books, The Random House, London, 2003.
Posted by at No comments:

Quantum Gravity and Dark Energy Using Fractal Planck Scaling

Quantum Gravity and Dark Energy Using Fractal Planck Scaling
Author(s)
L. Marek Crnjac, M. S. El Naschie
Following an inspiring idea due to D. Gross, we arrive at a topological Planck energy Ep and a corresponding topological Planck length  effectively scaling the Planck scale from esoterically large  and equally esoterically small  numbers to a manageably  where P(H) is the famous Hardy’s probability for quantum entanglement which amounts to almost 9 percent and  Based on these results, we conclude the equivalence of Einstein-Rosen “wormhole” bridges and Einstein’s Podolsky-Rosen’s spooky action at a distance. In turn these results are shown to be consistent with distinguishing two energy components which results in , namely the quantum zero set particle component  which we can measure and the quantum empty set wave component which we cannot measure , i.e. the missing dark energy. Together the two components add to  where E is the total energy, m is the mass and c is the speed of light. In other words, the present new derivation of the world’s most celebrated formula explains in one stroke the two most puzzling problems of quantum physics and relativistic cosmology, namely the physicomathematical meaning of the wave function and the nature of dark energy. In essence they are one and the same when looked upon from the view point of quantum-fractal geometry.
KEYWORDS
Scaling the Planck Scale; Quantum Entanglement; Dark Energy; Kaluza-Klein Space-Time; Worm Hole; Action at a Distance; Unruh Temperature; Hawking’s Negative Energy; Black Hole Physics; Cantorian Geometry; Fractals in Physics

To continue reading the paper please downloads it from here

Cite this paper
L. Crnjac and M. Naschie, "Quantum Gravity and Dark Energy Using Fractal Planck Scaling," Journal of Modern Physics, Vol. 4 No. 11A, 2013, pp. 31-38. doi: 10.4236/jmp.2013.411A1005.
[1]D. Gross, Physics Today, Vol. 42, 1989, p. 9.
http://dx.doi.org/10.1063/1.2811040
[2]M.S. El Naschie and Atef Helal, International Journal of Astronomy and Astrophysics, Vol. 3, 2013, pp. 318-343.
[3]M. S. El Naschie, Journal of Quantum Information Science, Vol. 1, 2011, pp. 50-53.
http://dx.doi.org/10.4236/jqis.2011.12007
[4]M. S. El Naschie, Journal of Quantum Information Science, Vol. 3, 2013, pp. 23-26.
http://dx.doi.org/10.4236/jqis.2013.31006
[5]M. S. El Naschie, Chaos, Solitons & Fractals, Vol. 19, 2004, pp. 209-236.
http://dx.doi.org/10.1016/S0960-0779(03)00278-9
[6]A. Elokaby, Chaos, Solitons & Fractals, Vol. 41, 2009, pp. 1616-1618.
http://dx.doi.org/10.1016/j.chaos.2008.07.003
[7]P. S. Addison, “Fractals and Chaos,” IOP, Bristol, 1997.
http://dx.doi.org/10.1887/0750304006
[8]F. Diacu and P. Holmes, “Celestial Encounters—The Origins of Chaos and Stability,” Princeton University Press, Princeton, 1996.
[9]M. S. El Naschie, International Journal of Modern Nonlinear Theory and Application, Vol. 2, 2013, pp. 107-121.
http://dx.doi.org/10.4236/ijmnta.2013.22014
[10]M. S. El Naschie, Journal of Quantum Information Science, Vol. 3, 2013, pp. 57-77.
http://dx.doi.org/10.4236/jqis.2013.32011
[11]A. Connes, “Non-Commutative Geometry,” Academic Press, New York, 1994.
[12]J. Bellissard, “Gap labeling theorems for Schrodinger operators,” In: M. Waldschmidt, P. Monsa, J. Luck and C. Itzykon, Eds., Chapter 12 in From Number Theory to Physics, Springer, Berlin, 1992.
[13]G. Landi, “An Introduction to Non-Commutative Space and Their Geometries,” Springer, Berlin, 1997.
[14]L. Marek-Crnjac, et al., International Journal of Modern Nonlinear Theory and Application, Vol. 2, 2013, pp. 78-88. http://dx.doi.org/10.4236/ijmnta.2013.21A010
[15]M. S. El Naschie and J.-H. He, Fractal Space-Time and Non-Commutative Geometry in High Energy Physics, Vol. 2, 2012, pp. 41-49.
[16]M. S. El Naschie, Fractal Space-Time and Non-Commutative Geometry in High Energy Physics, Vol. 2, 2012, pp. 135-142.
[17]M. S. El Naschie, J.-H. He, S. Nada, L. Marek-Crnjac and M. Atef Helal, Fractal Space-Time and Non-Commutative Geometry in High Energy Physics, Vol. 2, 2012, pp. 80-92.
[18]J.-H. He and M. S. El Naschie, Fractal Space-Time and Non-Commutative Geometry in High Energy Physics, Vol. 2, 2012, pp. 94-98.
[19]M. S. El Naschie and J.-H. He, Fractal Space-Time and Non-Commutative Geometry in High Energy Physics, Vol. 1, 2011, pp. 3-9.
[20]M. S. El Naschie and S. Olsen, Fractal Space-Time and Non-Commutative Geometry in High Energy Physics, Vol. 1, 2011, pp. 11-24.
[21]M. S. El Naschie and O. E. Rossler, Fractal Space-Time and Non-Commutative Geometry in High Energy Physics, Vol. 2, 2012, pp. 56-65.
[22]J.-H. He, T. Zhong, L. Xu, L. Marek-Crnjac, S. Nada and M. Atef Helal, Nonlinear Science Letters B, Vol. 1, 2011, pp. 15-24.
[23]M.S. El Naschie, Nonlinear Science Letters A, Vol. 2, 2011, pp. 1-9.
[24]M. S. El Naschie, L. Marek-Crnjac, J.-H. He and M. Atef Helal, Fractal Space-Time and Non-Commutative Geometry in High Energy Physics, Vol. 3, 2013, pp. 3-10.
[25]T. Zhong and J.-H. He, Fractal Space-Time and Non-Commutative Geometry in High Energy Physics, Vol. 3, 2013, pp. 46-49.
[26]M. S. El Naschie, Journal of Modern Physics, Vol. 4, 2013, pp. 757-760.
http://dx.doi.org/10.4236/jmp.2013.46103
[27]M. S. El Naschie, Journal of Modern Physics, Vol. 4, 2013, pp. 591-596.
http://dx.doi.org/10.4236/jmp.2013.45084
[28]M. S. El Naschie, Gravitation and Cosmology, Vol. 19, 2013, pp. 151-155.
http://dx.doi.org/10.1134/S0202289313030031
[29]S. Krantz and H. Parks, “Geometric Integration Theory,” Birkhauser, Boston, 2008.
http://dx.doi.org/10.1007/978-0-8176-4679-0
[30]R. Penrose, “The Road to Reality,” Johnathan Cape, London, 2004.
[31]L. Hardy, Physical Review Letters, Vol. 71, 1993, pp. 1665-1668.
http://dx.doi.org/10.1103/PhysRevLett.71.1665
[32]P. Kwiat and L. Hardy, American Journal of Physics, Vol. 68, 2000, p. 33. http://dx.doi.org/10.1119/1.19369
[33]N. D. Mermin, American Journal of Physics, Vol. 62, 1994, p. 880. http://dx.doi.org/10.1119/1.17733
[34]E. J. Copeland, M. Sami and S. Tsujikawa, “Dynamics of Dark Energy,” 2006. arXiv: hep-th/0603057V3
[35]S. Perlmutter, et al., The Astrophysical Journal, Vol. 517, 1999, pp. 565-585. http://dx.doi.org/10.1086/307221
[36]M. S. El Naschie, Chaos, Solitons & Fractals, Vol. 10, 1999, pp. 1807-1811.
http://dx.doi.org/10.1016/S0960-0779(99)00008-9
[37]M. S. El Naschie, Chaos, Solitons & Fractals, Vol. 14, 2002, pp. 1117-1120.
[38]M. S. El Naschie, Chaos, Solitons & Fractals, Vol. 9, 1998, pp. 1445-1471.
http://dx.doi.org/10.1016/S0960-0779(98)00120-9
[39]M. S. El Naschie, Chaos, Solitons & Fractals, Vol. 38, 2008, pp. 1260-1268.
http://dx.doi.org/10.1016/j.chaos.2008.07.010
[40]M. S. El Naschie, Chaos, Solitons & Fractals, Vol. 29, 2006, pp. 23-35.
http://dx.doi.org/10.1016/j.chaos.2005.11.079
[41]M. S. El Naschie, Chaos, Solitons & Fractals, Vol. 41, 2009, pp. 1799-1803.
http://dx.doi.org/10.1016/j.chaos.2008.07.025
[42]M. S. El Naschie, Chaos, Solitons & Fractals, Vol. 41, 2009, pp. 2787-2789.
http://dx.doi.org/10.1016/j.chaos.2008.10.011
[43]M. S. El Naschie, New Advances in Physics, Vol. 1, 2007, pp. 111-122.
[44]J. Mageuijo and L. Smolin, “Lorentz Invariance with an Invariant Energy Scale,” Cornell University Library, Ithaca, 2001. arXiv: hep-th/0112090V2
[45]T. Zhong, Chaos, Solitons & Fractals, Vol. 42, 2009, pp. 1780-1783. http://dx.doi.org/10.1016/j.chaos.2009.03.079
[46]M. S. El Naschie, Chaos, Solitons & Fractals, Vol. 14, 2002, pp. 1121-1126.
http://dx.doi.org/10.1016/S0960-0779(02)00172-8
[47]M. S. El Naschie, Chaos, Solitons & Fractals, Vol. 28, 2006, pp. 1366-1371.
http://dx.doi.org/10.1016/j.chaos.2005.11.001
[48]M. S. El Naschie, Chaos, Solitons & Fractals, Vol. 29, 2006, pp. 816-822.
http://dx.doi.org/10.1016/j.chaos.2006.01.013
[49]M. S. El Naschie, Chaos, Solitons & Fractals, Vol. 16, No. 2, 2003, pp. 353-366.
http://dx.doi.org/10.1016/S0960-0779(02)00440-X
[50]M. S. El Naschie, Chaos, Solitons & Fractals, Vol. 13, 2002, pp. 1167-1174.
http://dx.doi.org/10.1016/S0960-0779(01)00210-7
[51]M. S. El Naschie, Chaos, Solitons & Fractals, Vol. 13, 2002, pp. 1935-1945.
http://dx.doi.org/10.1016/S0960-0779(01)00242-9
[52]A. Pais, “Subtle Is the Lord: The Science and Life of Albert Einstein,” Oxford University Press, Oxford, 1982.
[53]J.-H. He and M. S. El Naschie, Fractal Space-Time and Non-Commutative Geometry in High Energy Physics, Vol. 3, 2013, pp. 59-62.
[54]A. Gefter, “The Infinity Illusion,” New Scientist, 17 August 2013, pp. 32-35.
[55]M. S. El Naschie, Chaos, Solitons & Fractals, Vol. 41, 2009, pp. 2635-2646.
http://dx.doi.org/10.1016/j.chaos.2008.09.059
[56]R. Elwes, “Ultimate logic,” New Scientist, 30 July 2011, pp. 30-33.
http://dx.doi.org/10.1016/S0262-4079(11)61838-1
[57]M. S. El Naschie, International Journal of Modern Nonlinear Theory and Application, Vol. 2, 2013, pp. 55-59.
[58]M. S. El Naschie, International Journal of Theoretical Physics, Vol. 37, 1998, pp. 2935-2951.
http://dx.doi.org/10.1023/A:1026679628582
[59]M. S. El Naschie, International Journal of Modern Physics E, Vol. 13, 2004, pp. 835-849.
http://dx.doi.org/10.1142/S0218301304002429
[60]M. S. El Naschie, “From Hilbert Space to the Number of Higgs Particles via the Quantum Two-Slit Experiment,” In: P. Weibel, G. Ord and O. Rossler, Eds., Space-Time Physics and Fractality, Festshrift in Honour of Mohamed El Naschie, Springer Verlag, Wien and New York, 2005, pp. 223-231. http://dx.doi.org/10.1007/3-211-37848-0_14
[61]M. S. El Naschie, Open Journal of Microphysics, Vol. 3, 2013, pp. 64-70.
http://dx.doi.org/10.4236/ojm.2013.33012
[62]E. Goldfain, Chaos, Solitons & Fractals, Vol. 20, 2004, pp. 427-435.
http://dx.doi.org/10.1016/j.chaos.2003.10.012
[63]G. N. Ord, Annals of Physics, Vol. 324, 2009, pp. 1211-1218.
[64]M. S. El Naschie, Chaos, Solitons & Fractals, Vol. 25, 2005, pp. 521-524.
http://dx.doi.org/10.1016/j.chaos.2005.01.022
[65]A. Elokaby, Chaos, Solitons & Fractals, Vol. 42, 2009, pp. 303-305.
http://dx.doi.org/10.1016/j.chaos.2008.12.001
[66]G. Iovane and S. Nada, Chaos, Solitons & Fractals, Vol. 41, 2009, pp. 641-642.
http://dx.doi.org/10.1016/j.chaos.2008.11.024
[67]J.-H. He, E. Goldfain, L. D. Sigalotti and A. Mejias, “Beyond the 2006 Physics Nobel Prize for COBE,” China Culture & Scientific Publishing, 2006.
[68]M. S. El Naschie, Journal of Modern Physics, Vol. 4, 2013, pp. 354-356.
http://dx.doi.org/10.4236/jmp.2013.43049
[69]D. R. Finkelstein, “Quantum Relativity,” Springer, Berlin, 1996. http://dx.doi.org/10.1007/978-3-642-60936-7
[70]C. Rovelli, “Quantum Gravity,” Cambridge Press, Cambridge, 2004.
[71]Y. Gnedin, A. Grib and V. Matepanenko, “Proceedings of the Third Alexander Friedmann International Seminar on Gravitation and Cosmology,” Friedmann Lab. Pub., St. Petersberg, 1995.
[72]A. Vileukin and E. Shellard, “Cosmic Strings and Other Topological Defects,” Cambridge University Press, Cambridge, 2001.
Posted by at No comments:
Subscribe to: Posts (Atom)