Quantum Gravity and Dark Energy Using Fractal Planck Scaling
To continue reading the paper please downloads it from here
Author(s)
L. Marek Crnjac, M. S. El Naschie
Following an inspiring idea due to D. Gross, we arrive at a topological Planck energy Ep and a corresponding topological Planck length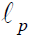 
effectively scaling the Planck scale from esoterically large 
and equally esoterically small 
numbers to a manageably 
where P(H) is the famous Hardy’s probability for quantum entanglement which amounts to almost 9 percent and 
Based on these results, we conclude the equivalence of Einstein-Rosen “wormhole” bridges and Einstein’s Podolsky-Rosen’s spooky action at a distance. In turn these results are shown to be consistent with distinguishing two energy components which results in 
, namely the quantum zero set particle component 
which we can measure and the quantum empty set wave component which we cannot measure 
, i.e. the missing dark energy. Together the two components add to 
where E is the total energy, m is the mass and c is the speed of light. In other words, the present new derivation of the world’s most celebrated formula explains in one stroke the two most puzzling problems of quantum physics and relativistic cosmology, namely the physicomathematical meaning of the wave function and the nature of dark energy. In essence they are one and the same when looked upon from the view point of quantum-fractal geometry.
effectively scaling the Planck scale from esoterically large 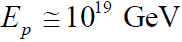and equally esoterically small 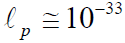numbers to a manageably 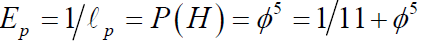where P(H) is the famous Hardy’s probability for quantum entanglement which amounts to almost 9 percent and 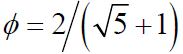Based on these results, we conclude the equivalence of Einstein-Rosen “wormhole” bridges and Einstein’s Podolsky-Rosen’s spooky action at a distance. In turn these results are shown to be consistent with distinguishing two energy components which results in 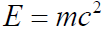, namely the quantum zero set particle component 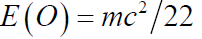which we can measure and the quantum empty set wave component which we cannot measure 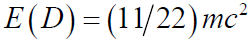, i.e. the missing dark energy. Together the two components add to 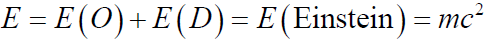where E is the total energy, m is the mass and c is the speed of light. In other words, the present new derivation of the world’s most celebrated formula explains in one stroke the two most puzzling problems of quantum physics and relativistic cosmology, namely the physicomathematical meaning of the wave function and the nature of dark energy. In essence they are one and the same when looked upon from the view point of quantum-fractal geometry.
KEYWORDS
Scaling the Planck Scale; Quantum Entanglement; Dark Energy; Kaluza-Klein Space-Time; Worm Hole; Action at a Distance; Unruh Temperature; Hawking’s Negative Energy; Black Hole Physics; Cantorian Geometry; Fractals in Physics
Cite this paper
L. Crnjac and M. Naschie, "Quantum Gravity and Dark Energy Using Fractal Planck Scaling," Journal of Modern Physics, Vol. 4 No. 11A, 2013, pp. 31-38. doi: 10.4236/jmp.2013.411A1005.
| [1] | D. Gross, Physics Today, Vol. 42, 1989, p. 9. http://dx.doi.org/10.1063/1.2811040 |
| [2] | M.S. El Naschie and Atef Helal, International Journal of Astronomy and Astrophysics, Vol. 3, 2013, pp. 318-343. |
| [3] | M. S. El Naschie, Journal of Quantum Information Science, Vol. 1, 2011, pp. 50-53. http://dx.doi.org/10.4236/jqis.2011.12007 |
| [4] | M. S. El Naschie, Journal of Quantum Information Science, Vol. 3, 2013, pp. 23-26. http://dx.doi.org/10.4236/jqis.2013.31006 |
| [5] | M. S. El Naschie, Chaos, Solitons & Fractals, Vol. 19, 2004, pp. 209-236. http://dx.doi.org/10.1016/S0960-0779(03)00278-9 |
| [6] | A. Elokaby, Chaos, Solitons & Fractals, Vol. 41, 2009, pp. 1616-1618. http://dx.doi.org/10.1016/j.chaos.2008.07.003 |
| [7] | P. S. Addison, “Fractals and Chaos,” IOP, Bristol, 1997. http://dx.doi.org/10.1887/0750304006 |
| [8] | F. Diacu and P. Holmes, “Celestial Encounters—The Origins of Chaos and Stability,” Princeton University Press, Princeton, 1996. |
| [9] | M. S. El Naschie, International Journal of Modern Nonlinear Theory and Application, Vol. 2, 2013, pp. 107-121. http://dx.doi.org/10.4236/ijmnta.2013.22014 |
| [10] | M. S. El Naschie, Journal of Quantum Information Science, Vol. 3, 2013, pp. 57-77. http://dx.doi.org/10.4236/jqis.2013.32011 |
| [11] | A. Connes, “Non-Commutative Geometry,” Academic Press, New York, 1994. |
| [12] | J. Bellissard, “Gap labeling theorems for Schrodinger operators,” In: M. Waldschmidt, P. Monsa, J. Luck and C. Itzykon, Eds., Chapter 12 in From Number Theory to Physics, Springer, Berlin, 1992. |
| [13] | G. Landi, “An Introduction to Non-Commutative Space and Their Geometries,” Springer, Berlin, 1997. |
| [14] | L. Marek-Crnjac, et al., International Journal of Modern Nonlinear Theory and Application, Vol. 2, 2013, pp. 78-88. http://dx.doi.org/10.4236/ijmnta.2013.21A010 |
| [15] | M. S. El Naschie and J.-H. He, Fractal Space-Time and Non-Commutative Geometry in High Energy Physics, Vol. 2, 2012, pp. 41-49. |
| [16] | M. S. El Naschie, Fractal Space-Time and Non-Commutative Geometry in High Energy Physics, Vol. 2, 2012, pp. 135-142. |
| [17] | M. S. El Naschie, J.-H. He, S. Nada, L. Marek-Crnjac and M. Atef Helal, Fractal Space-Time and Non-Commutative Geometry in High Energy Physics, Vol. 2, 2012, pp. 80-92. |
| [18] | J.-H. He and M. S. El Naschie, Fractal Space-Time and Non-Commutative Geometry in High Energy Physics, Vol. 2, 2012, pp. 94-98. |
| [19] | M. S. El Naschie and J.-H. He, Fractal Space-Time and Non-Commutative Geometry in High Energy Physics, Vol. 1, 2011, pp. 3-9. |
| [20] | M. S. El Naschie and S. Olsen, Fractal Space-Time and Non-Commutative Geometry in High Energy Physics, Vol. 1, 2011, pp. 11-24. |
| [21] | M. S. El Naschie and O. E. Rossler, Fractal Space-Time and Non-Commutative Geometry in High Energy Physics, Vol. 2, 2012, pp. 56-65. |
| [22] | J.-H. He, T. Zhong, L. Xu, L. Marek-Crnjac, S. Nada and M. Atef Helal, Nonlinear Science Letters B, Vol. 1, 2011, pp. 15-24. |
| [23] | M.S. El Naschie, Nonlinear Science Letters A, Vol. 2, 2011, pp. 1-9. |
| [24] | M. S. El Naschie, L. Marek-Crnjac, J.-H. He and M. Atef Helal, Fractal Space-Time and Non-Commutative Geometry in High Energy Physics, Vol. 3, 2013, pp. 3-10. |
| [25] | T. Zhong and J.-H. He, Fractal Space-Time and Non-Commutative Geometry in High Energy Physics, Vol. 3, 2013, pp. 46-49. |
| [26] | M. S. El Naschie, Journal of Modern Physics, Vol. 4, 2013, pp. 757-760. http://dx.doi.org/10.4236/jmp.2013.46103 |
| [27] | M. S. El Naschie, Journal of Modern Physics, Vol. 4, 2013, pp. 591-596. http://dx.doi.org/10.4236/jmp.2013.45084 |
| [28] | M. S. El Naschie, Gravitation and Cosmology, Vol. 19, 2013, pp. 151-155. http://dx.doi.org/10.1134/S0202289313030031 |
| [29] | S. Krantz and H. Parks, “Geometric Integration Theory,” Birkhauser, Boston, 2008. http://dx.doi.org/10.1007/978-0-8176-4679-0 |
| [30] | R. Penrose, “The Road to Reality,” Johnathan Cape, London, 2004. |
| [31] | L. Hardy, Physical Review Letters, Vol. 71, 1993, pp. 1665-1668. http://dx.doi.org/10.1103/PhysRevLett.71.1665 |
| [32] | P. Kwiat and L. Hardy, American Journal of Physics, Vol. 68, 2000, p. 33. http://dx.doi.org/10.1119/1.19369 |
| [33] | N. D. Mermin, American Journal of Physics, Vol. 62, 1994, p. 880. http://dx.doi.org/10.1119/1.17733 |
| [34] | E. J. Copeland, M. Sami and S. Tsujikawa, “Dynamics of Dark Energy,” 2006. arXiv: hep-th/0603057V3 |
| [35] | S. Perlmutter, et al., The Astrophysical Journal, Vol. 517, 1999, pp. 565-585. http://dx.doi.org/10.1086/307221 |
| [36] | M. S. El Naschie, Chaos, Solitons & Fractals, Vol. 10, 1999, pp. 1807-1811. http://dx.doi.org/10.1016/S0960-0779(99)00008-9 |
| [37] | M. S. El Naschie, Chaos, Solitons & Fractals, Vol. 14, 2002, pp. 1117-1120. |
| [38] | M. S. El Naschie, Chaos, Solitons & Fractals, Vol. 9, 1998, pp. 1445-1471. http://dx.doi.org/10.1016/S0960-0779(98)00120-9 |
| [39] | M. S. El Naschie, Chaos, Solitons & Fractals, Vol. 38, 2008, pp. 1260-1268. http://dx.doi.org/10.1016/j.chaos.2008.07.010 |
| [40] | M. S. El Naschie, Chaos, Solitons & Fractals, Vol. 29, 2006, pp. 23-35. http://dx.doi.org/10.1016/j.chaos.2005.11.079 |
| [41] | M. S. El Naschie, Chaos, Solitons & Fractals, Vol. 41, 2009, pp. 1799-1803. http://dx.doi.org/10.1016/j.chaos.2008.07.025 |
| [42] | M. S. El Naschie, Chaos, Solitons & Fractals, Vol. 41, 2009, pp. 2787-2789. http://dx.doi.org/10.1016/j.chaos.2008.10.011 |
| [43] | M. S. El Naschie, New Advances in Physics, Vol. 1, 2007, pp. 111-122. |
| [44] | J. Mageuijo and L. Smolin, “Lorentz Invariance with an Invariant Energy Scale,” Cornell University Library, Ithaca, 2001. arXiv: hep-th/0112090V2 |
| [45] | T. Zhong, Chaos, Solitons & Fractals, Vol. 42, 2009, pp. 1780-1783. http://dx.doi.org/10.1016/j.chaos.2009.03.079 |
| [46] | M. S. El Naschie, Chaos, Solitons & Fractals, Vol. 14, 2002, pp. 1121-1126. http://dx.doi.org/10.1016/S0960-0779(02)00172-8 |
| [47] | M. S. El Naschie, Chaos, Solitons & Fractals, Vol. 28, 2006, pp. 1366-1371. http://dx.doi.org/10.1016/j.chaos.2005.11.001 |
| [48] | M. S. El Naschie, Chaos, Solitons & Fractals, Vol. 29, 2006, pp. 816-822. http://dx.doi.org/10.1016/j.chaos.2006.01.013 |
| [49] | M. S. El Naschie, Chaos, Solitons & Fractals, Vol. 16, No. 2, 2003, pp. 353-366. http://dx.doi.org/10.1016/S0960-0779(02)00440-X |
| [50] | M. S. El Naschie, Chaos, Solitons & Fractals, Vol. 13, 2002, pp. 1167-1174. http://dx.doi.org/10.1016/S0960-0779(01)00210-7 |
| [51] | M. S. El Naschie, Chaos, Solitons & Fractals, Vol. 13, 2002, pp. 1935-1945. http://dx.doi.org/10.1016/S0960-0779(01)00242-9 |
| [52] | A. Pais, “Subtle Is the Lord: The Science and Life of Albert Einstein,” Oxford University Press, Oxford, 1982. |
| [53] | J.-H. He and M. S. El Naschie, Fractal Space-Time and Non-Commutative Geometry in High Energy Physics, Vol. 3, 2013, pp. 59-62. |
| [54] | A. Gefter, “The Infinity Illusion,” New Scientist, 17 August 2013, pp. 32-35. |
| [55] | M. S. El Naschie, Chaos, Solitons & Fractals, Vol. 41, 2009, pp. 2635-2646. http://dx.doi.org/10.1016/j.chaos.2008.09.059 |
| [56] | R. Elwes, “Ultimate logic,” New Scientist, 30 July 2011, pp. 30-33. http://dx.doi.org/10.1016/S0262-4079(11)61838-1 |
| [57] | M. S. El Naschie, International Journal of Modern Nonlinear Theory and Application, Vol. 2, 2013, pp. 55-59. |
| [58] | M. S. El Naschie, International Journal of Theoretical Physics, Vol. 37, 1998, pp. 2935-2951. http://dx.doi.org/10.1023/A:1026679628582 |
| [59] | M. S. El Naschie, International Journal of Modern Physics E, Vol. 13, 2004, pp. 835-849. http://dx.doi.org/10.1142/S0218301304002429 |
| [60] | M. S. El Naschie, “From Hilbert Space to the Number of Higgs Particles via the Quantum Two-Slit Experiment,” In: P. Weibel, G. Ord and O. Rossler, Eds., Space-Time Physics and Fractality, Festshrift in Honour of Mohamed El Naschie, Springer Verlag, Wien and New York, 2005, pp. 223-231. http://dx.doi.org/10.1007/3-211-37848-0_14 |
| [61] | M. S. El Naschie, Open Journal of Microphysics, Vol. 3, 2013, pp. 64-70. http://dx.doi.org/10.4236/ojm.2013.33012 |
| [62] | E. Goldfain, Chaos, Solitons & Fractals, Vol. 20, 2004, pp. 427-435. http://dx.doi.org/10.1016/j.chaos.2003.10.012 |
| [63] | G. N. Ord, Annals of Physics, Vol. 324, 2009, pp. 1211-1218. |
| [64] | M. S. El Naschie, Chaos, Solitons & Fractals, Vol. 25, 2005, pp. 521-524. http://dx.doi.org/10.1016/j.chaos.2005.01.022 |
| [65] | A. Elokaby, Chaos, Solitons & Fractals, Vol. 42, 2009, pp. 303-305. http://dx.doi.org/10.1016/j.chaos.2008.12.001 |
| [66] | G. Iovane and S. Nada, Chaos, Solitons & Fractals, Vol. 41, 2009, pp. 641-642. http://dx.doi.org/10.1016/j.chaos.2008.11.024 |
| [67] | J.-H. He, E. Goldfain, L. D. Sigalotti and A. Mejias, “Beyond the 2006 Physics Nobel Prize for COBE,” China Culture & Scientific Publishing, 2006. |
| [68] | M. S. El Naschie, Journal of Modern Physics, Vol. 4, 2013, pp. 354-356. http://dx.doi.org/10.4236/jmp.2013.43049 |
| [69] | D. R. Finkelstein, “Quantum Relativity,” Springer, Berlin, 1996. http://dx.doi.org/10.1007/978-3-642-60936-7 |
| [70] | C. Rovelli, “Quantum Gravity,” Cambridge Press, Cambridge, 2004. |
| [71] | Y. Gnedin, A. Grib and V. Matepanenko, “Proceedings of the Third Alexander Friedmann International Seminar on Gravitation and Cosmology,” Friedmann Lab. Pub., St. Petersberg, 1995. |
| [72] | A. Vileukin and E. Shellard, “Cosmic Strings and Other Topological Defects,” Cambridge University Press, Cambridge, 2001. |
No comments:
Post a Comment