Nash Embedding of Witten’s M-Theory and the Hawking-Hartle Quantum Wave of Dark Energy
Author(s)
Mohamed S. El Naschie
Euclidean embedding of the 11-dimensional M-theory turned out to require a very large space leaving lavish amounts of 242 dimensional pseudo truly empty “regions” devoid of space and time and consequently of anything resembling ordinary physical energy density. It is shown here using Nash embedding that the ratio of “solid” M-theory spacetime to its required embedding “non-spacetime” is 1/22 for a classical theory and1/22.18033989 for an analogous fractal theory. This then leads to a maximal ordinary energy density equation equal to that of Einstein’s famous formula E=mc2 but multiplied with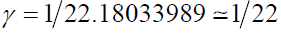 
in full agreement with previous results obtained using relatively more conventional methods including running the electromagnetic fine structure constant in the exact solution of the hydrogen atom. Consequently, the new equation corresponds to a quantum relativity theory which unlike Einstein’s original equation gives quantitative predictions which agree perfectly with the cosmological measurements of WMAP and the analysis of certain supernova events. Never the less in our view dark energy also exists being the energy of the quantum wave amounting to 95.5 present of the total Einstein theoretical energy which is blind to any distinction between ordinary energy of the quantum particle and the dark energy of the quantum wave. However, since measurement leads to the collapse of the Hawking-Hartle quantum wave, dark energy being a quantum wave non-ordinary energy could not possibly be measured in the usual way unless highly refined quantum wave non-demolition technology is developed if possible. It is a further reason that dark energy having a different sign to ordinary energy is the cause behind the anti gravity force which is pushing the universe apart and accelerating cosmic expansion. Consequently it can be seen as the result of anticlastic Cartan-like curvature caused by extra compactified dimensions of spacetime. A simple toy model demonstration of the effect of curvature in a “material” space is briefly discussed.
in full agreement with previous results obtained using relatively more conventional methods including running the electromagnetic fine structure constant in the exact solution of the hydrogen atom. Consequently, the new equation corresponds to a quantum relativity theory which unlike Einstein’s original equation gives quantitative predictions which agree perfectly with the cosmological measurements of WMAP and the analysis of certain supernova events. Never the less in our view dark energy also exists being the energy of the quantum wave amounting to 95.5 present of the total Einstein theoretical energy which is blind to any distinction between ordinary energy of the quantum particle and the dark energy of the quantum wave. However, since measurement leads to the collapse of the Hawking-Hartle quantum wave, dark energy being a quantum wave non-ordinary energy could not possibly be measured in the usual way unless highly refined quantum wave non-demolition technology is developed if possible. It is a further reason that dark energy having a different sign to ordinary energy is the cause behind the anti gravity force which is pushing the universe apart and accelerating cosmic expansion. Consequently it can be seen as the result of anticlastic Cartan-like curvature caused by extra compactified dimensions of spacetime. A simple toy model demonstration of the effect of curvature in a “material” space is briefly discussed.
KEYWORDS
Nash Euclidean Embedding; Quantum Entanglement; Dark Energy of the Quantum Wave; Quantum Gravity; Ordinary Energy of the Quantum Particle; Hawking-Hartle Wave of the Cosmos; Quantum Wave Non-Demolition; Witten’s M-Theory
Cite this paper
M. Naschie, "Nash Embedding of Witten’s M-Theory and the Hawking-Hartle Quantum Wave of Dark Energy," Journal of Modern Physics, Vol. 4 No. 10, 2013, pp. 1417-1428. doi: 10.4236/jmp.2013.410170.
| [1] | J. Nash, Annals of Mathematics, Vol. 63, 1956, p. 20. http://dx.doi.org/10.2307/1969989 |
| [2] | J. Nash, Koninklijke Nederlandse Akademie van Wetenschappen, Vol. 58, 1955, p. 545. |
| [3] | M. S. El Naschie, Chaos, Solitons & Fractals, Vol. 18, 2003, pp. 635-641. http://dx.doi.org/10.1016/S0960-0779(03)00007-9 |
| [4] | R. Penrose, “The Road to Reality. A Complete Guide to the Laws of the Universe,” Jonathan Cape, London, 2004. |
| [5] | W. Rindler, “Relativity,” Oxford Science Publications, Oxford, 2004. |
| [6] | M. S. El Naschie and L. Marek-Crnjac, International Journal of Modern Nonlinear Theory and Application, Vol. 1, 2012, pp. 118-124. http://dx.doi.org/10.4236/ijmnta.2012.14018 |
| [7] | E. J. Copeland, M. Sami and S. Tsujikawa, “Dynamics of Dark Energy,” 2006. arXiv: hep-th/0603057 |
| [8] | L. Amendola and S. Tsujikawa, “Dark Energy: Theory and Observations,” Cambridge University Press, Cambridge, 2010. http://dx.doi.org/10.1017/CBO9780511750823 |
| [9] | M. S. El Naschie, Chaos, Solitons & Fractals, Vol. 41, 2009, p. 2635. http://dx.doi.org/10.1016/j.chaos.2008.09.059 |
| [10] | M. S. El Naschie, M. S. El Naschie, Chaos, Solitons & Fractals, Vol. 19, 2004, p. 209. http://dx.doi.org/10.1016/S0960-0779(03)00278-9 |
| [11] | M. S. El Naschie, Chaos, Solitons & Fractals, Vol. 30, 2006, p. 579. http://dx.doi.org/10.1016/j.chaos.2006.03.030 |
| [12] | J. Polchinski, “String Theory,” Cambridge University Press, Cambridge, 1998. |
| [13] | M. S. El Naschie, International Journal of Nonlinear Sciences and Numerical Simulation, Vol. 7, 2006, p. 477. |
| [14] | M. S. El Naschie, International Journal of Nonlinear Sciences and Numerical Simulation, Vol. 7, 2006, p. 407. |
| [15] | M. S. El Naschie, L. Marek-Crnjac and J.-H. He, Fractal Spacetime & Noncommutative Geometry in Quantum & High Energy Physics, Vol. 2, 2012, p. 107. |
| [16] | M. Duff, International Journal of Modern Physics A, Vol. 11, 1996, p. 5623. http://dx.doi.org/10.1142/S0217751X96002583 |
| [17] | L. Marek-Crnjac, J.-H. He and M. S. El Naschie, Fractal Spacetime & Noncommutative Geometry in Quantum & High Energy Physics, Vol. 2, 2012, p. 118. |
| [18] | S. Nakajima, et al., “Foundations of Quantum Mechanics in The Light of New Technologies,” World Scientific, Singapore City, 1996. |
| [19] | L. Marek-Crnjac, et al., International Journal of Modern Nonlinear Theory and Application, Vol. 2, 2013, p. 78. |
| [20] | M.S. El Naschie, Journal of Quantum Information Science, Vol. 3, 2013, p. 23. http://dx.doi.org/10.4236/jqis.2013.31006 |
| [21] | L. Marek-Crnjac and J.-H. He, Fractal Spacetime and Noncommutative Geometry in Quantum and High Energy Physics, Vol. 2, 2012, p. 66. |
| [22] | V Braginsky and S. P. Vyatchanin, Doklady Akademii Nauk SSSR, Vol. 259, 1981, p. 570. [Soviet Physics— Doklady, Vol. 27, 1982, p. 478]. |
| [23] | M. S. El Naschie, Journal of Modern Physics, Vol. 4, 2013, p. 591. http://dx.doi.org/10.4236/jmp.2013.45084 |
| [24] | M. S. El Naschie, International Journal of Modern Nonlinear Theory and Application, Vol. 2, 2013, p. 43. http://dx.doi.org/10.4236/ijmnta.2013.21005 |
| [25] | M.S. El Naschie, Journal of Quantum Information Science, Vol. 3, 2013, p. 57. http://dx.doi.org/10.4236/jqis.2013.32011 |
| [26] | P. S. Wesson, “Five Dimensional Physics,” World Scientific, Singapore City, 2006. |
| [27] | M. S. El Naschie, “Stress, Stability and Chaos in Structural Engineering,” McGraw Hill, London, 1990. |
| [28] | M. S. El Naschie, International Journal of Modern Nonlinear Theory and Application, Vol. 2, 2013, pp. 107-121. http://dx.doi.org/10.4236/ijmnta.2013.22014 |
| [29] | M. S. El Naschie, Chaos, Solitons & Fractals, Vol. 23, 2005, pp. 1511-1514. http://dx.doi.org/10.1016/j.chaos.2004.08.008 |
| [30] | M. S. El Naschie, Chaos, Solitons & Fractals, Vol. 23, 2005, pp. 1931-1933. http://dx.doi.org/10.1016/j.chaos.2004.08.004 |
| [31] | R. Calella, A. W. Overhauser and S. A. Werner, Physical Review Letters, Vol. 34, 1975, pp. 1472-1474. http://dx.doi.org/10.1103/PhysRevLett.34.1472 |
| [32] | V. V. Nesvizhevky and A. K. Petukhov, The European Physical Journal C, Vol. 40, 2005, pp. 479-491. http://dx.doi.org/10.1140/epjc/s2005-02135-y |
| [33] | M. Reza Pahlavani, H. Rahbar and M. Ghezelbash, Open Journal of Microphysics, Vol. 3, 2013, pp. 1-7. http://dx.doi.org/10.4236/ojm.2013.31001 |
| [34] | J. Magueijo and J. W. Moffat, “Comments on ‘Note on Varying Speed of Light Theories’,” 2007. arXiv: 0705.4507 |
| [35] | J. Magueijo, “Faster Than the Speed of Light,” Arrow Books, The Random House, London, 2003. |
No comments:
Post a Comment